Morris Kline Quote

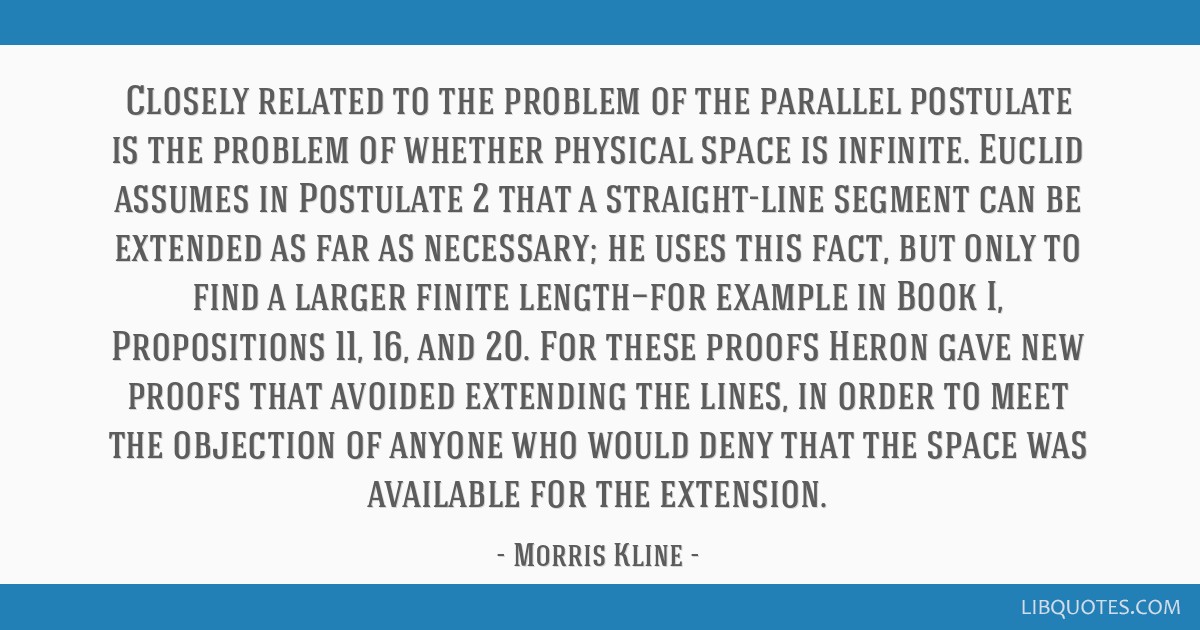
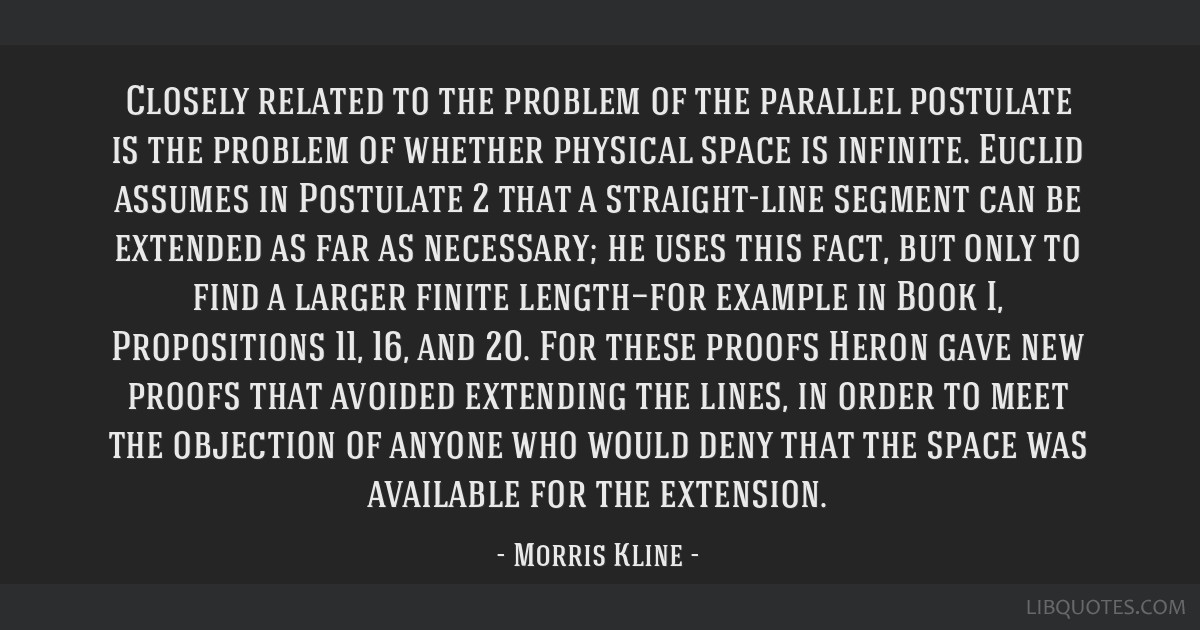
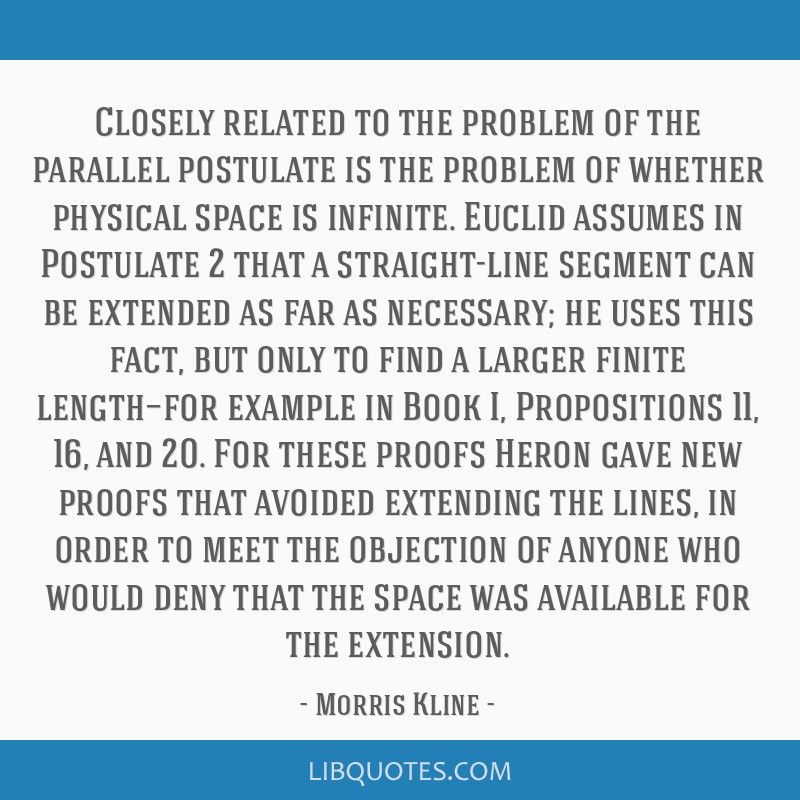
Closely related to the problem of the parallel postulate is the problem of whether physical space is infinite. Euclid assumes in Postulate 2 that a straight-line segment can be extended as far as necessary; he uses this fact, but only to find a larger finite length—for example in Book I, Propositions 11, 16, and 20. For these proofs Heron gave new proofs that avoided extending the lines, in order to meet the objection of anyone who would deny that the space was available for the extension.

p. 177 - Mathematical Thought from Ancient to Modern Times (1972)

Closely related to the problem of the parallel postulate is the problem of whether physical space is infinite. Euclid assumes in Postulate 2 that a...

Closely related to the problem of the parallel postulate is the problem of whether physical space is infinite. Euclid assumes in Postulate 2 that a...

Closely related to the problem of the parallel postulate is the problem of whether physical space is infinite. Euclid assumes in Postulate 2 that a...

Closely related to the problem of the parallel postulate is the problem of whether physical space is infinite. Euclid assumes in Postulate 2 that a...