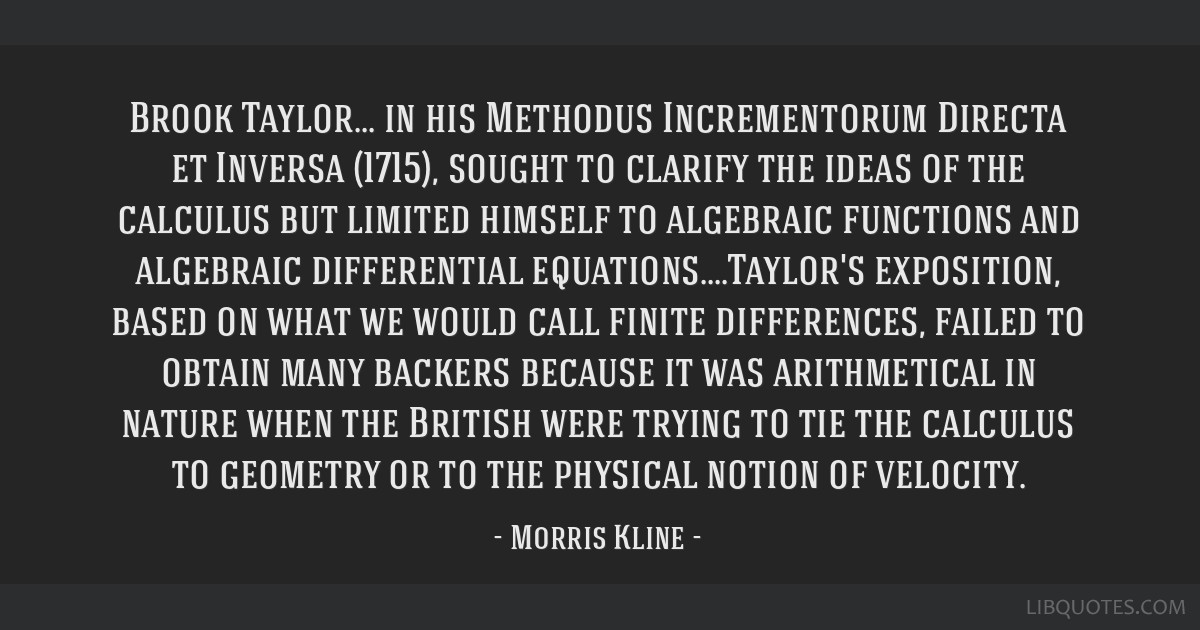
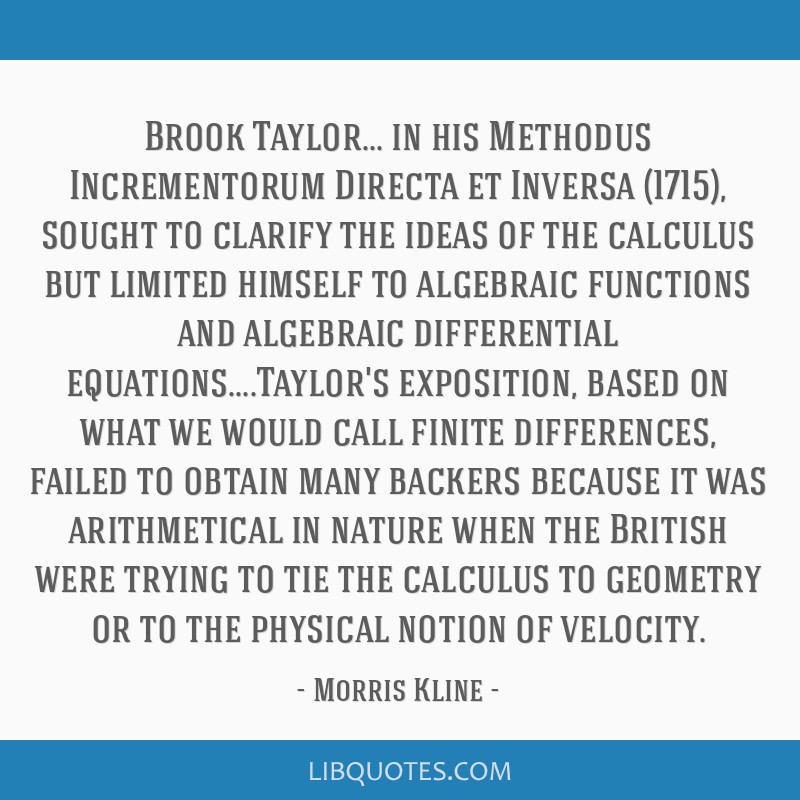
Brook Taylor... in his Methodus Incrementorum Directa et Inversa (1715), sought to clarify the ideas of the calculus but limited himself to algebraic functions and algebraic differential equations....Taylor's exposition, based on what we would call finite differences, failed to obtain many backers because it was arithmetical in nature when the British were trying to tie the calculus to geometry or to the physical notion of velocity.

p. 427 - Mathematical Thought from Ancient to Modern Times (1972)