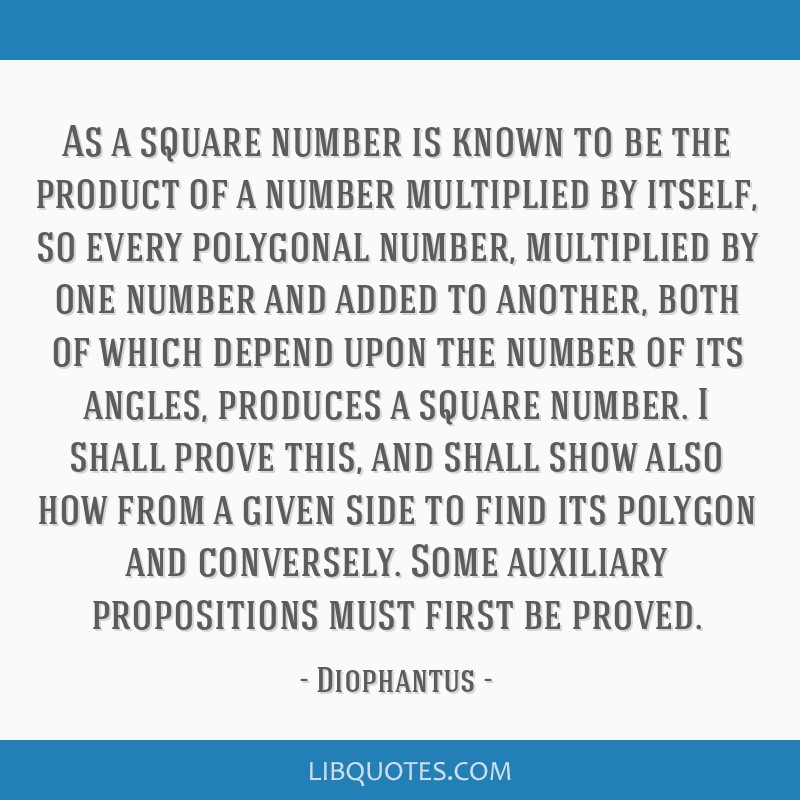
As a square number is known to be the product of a number multiplied by itself, so every polygonal number, multiplied by one number and added to another, both of which depend upon the number of its angles, produces a square number. I shall prove this, and shall show also how from a given side to find its polygon and conversely. Some auxiliary propositions must first be proved.

As quoted by James Gow, A Short History of Greek Mathematics (1884) - Arithmetica (c. 250 AD)

https://books.google.com/books?id=9d8DAAAAMAAJ